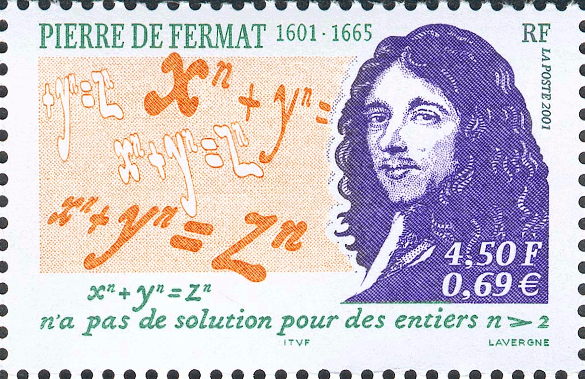
フェルマーの最終定理とは、3 以上の自然数 n について、xn + yn = zn となる 0 でない自然数 (x, y, z) の組み合わせが存在しない、という定理のことです。
フェルマーが驚くべき証明を得たと書き残したと伝えられ、長らく証明も反例もなされなかったことからフェルマー予想とも称されていましたが、360年後にアンドリュー・ワイルズによって完全に証明され、18年前、1995年の今日2月13日に誤りがないことが確認され、ワイルズの定理あるいはフェルマー・ワイルズの定理とも呼ばれるようになりました。
En matematiko, Lasta teoremo de Fermat deklaras, ke ne tri supernulaj entjeroj x, y, kaj z povas kontentigi la ekvacion xn + yn = zn por iu ajn entjero n pli granda ol 2. Tiun ĉi teoremon Pierre de Fermat unuafoje divenas en 1637, en la margino de kopio de Arithmetica kie li asertis ke, li havis pruvon sed ne povis skribi en la margino ĉar ĝi estis tro longa. Ne sukcesa pruvo estis eldonita ĝis 1995 malgraŭ la klopodoj de multaj matematikistoj.
En teoría de números, el último teorema de Fermat, o teorema de Fermat-Wiles, es uno de los teoremas más famosos en la historia de la matemática. Utilizando la notación moderna, se puede enunciar de la siguiente manera:
Si n es un número entero mayor que 2, entonces no existen números enteros x, y y z, tales que se cumpla la igualdad:
xn + yn = zn, Pierre de Fermat
Nótese que n es un entero mayor que 2, y x, y, z, no nulos. Es decir, ni x=0, ni y=0, ni z=0.
El teorema fue conjeturado por Pierre de Fermat en 1637, pero no fue demostrado hasta 1995 por Andrew Wiles ayudado por el matemático Richard Taylor. La búsqueda de una demostración estimuló el desarrollo de la teoría algebraica de números en el siglo XIX y la demostración del teorema de la modularidad en el siglo XX.
The proof was made for some values of n (like n=3, n=4, n=5 and n=7). Fermat, Euler, Sophie Germain, and other people did this.
However, the full proof must show that the equation has no solution for all values of n (when n is a whole number bigger than 2). The proof was very difficult to find, and Fermat's Last Theorem needed lots of time to be solved.
An English mathematician named Andrew Wiles found a solution in 1995, 358 years after Fermat wrote about it. Richard Taylor helped him find the solution. The proof took seven years of research.
After a few years of debate, people agreed that Andrew Wiles had solved the problem. Andrew Wiles used a lot of modern mathematics and even created new math when he made his solution. This mathematics was unknown when Fermat wrote his famous note, so Fermat could not have used it. This leads one to believe that Fermat did not in fact have a complete solution of the problem.
ご意見、ご質問等ございましたら、
<ernestotaju@yahoo.co.jp> へ。
<ernestotaju@yahoo.co.jp> へ。
ハヤカワ文庫 NF 282 〈数理を愉しむ〉シリーズ【1000円以上送料無料】天才数学者たちが挑ん... |
フェルマーの最終定理 ピュタゴラスに始まり、ワイルズが証明するまで/サイモン・シン/青木... |